RESEARCH INTERESTS
My research interests are varied but centre around the application of the methods of complex analysis to problems arising in the physical sciences and mathematical physics.
Among my areas of interest are:
- vortex dynamics
- free surface problems
- ideal hydrodynamics
- slow viscous (Stokes) flow
- the theory of quadrature domains and applications
- integrable systems theory
- automorphic functions
- potential theory and applications
- techniques of conformal mapping
- applications of algebraic geometry
Vortex motion in complex domains: here is a poster (which won First Prize at a recent Graduate Fair at Imperial College London) by my Ph.D student J.S. Marshall. It summarizes our joint work on the application of classical function theory to vortex motion around topography.
Click here to download a PDF version of my presentation at the 2004 American Physical Society meeting in Seattle. This gives a few more details on the mathematics of vortex motion in the presence of solid boundaries.
Generalized Stuart vortices
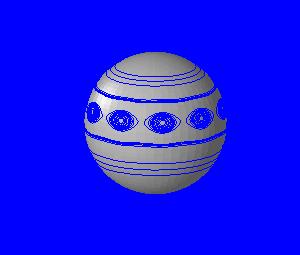
The above figure shows new solutions for Stuart vortices on a sphere (read more). Here is a poster giving a brief synopsis of the mathematical derivation of the solutions.
Ideal flow theory:
Click here to download a PDF version of my presentation at the 2005 American Physical Society meeting in Chicago. It concerns the calculation of the lift forces on multiple aerofoils.
Quadrature domains and applications:
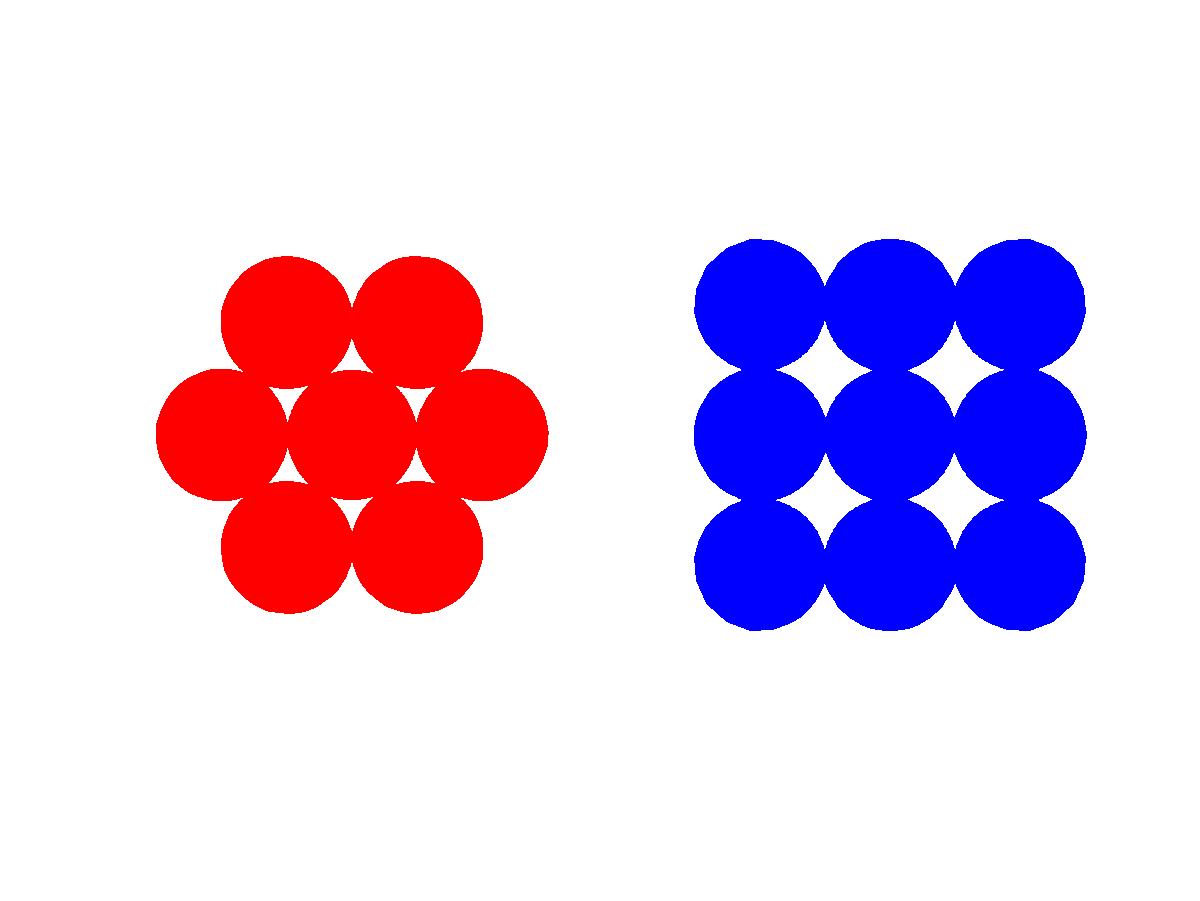
The above figure shows examples of two multiply connected quadrature domains; this is a class of planar domains which have important special properties with respect to the integration of analytic functions over their support. The domains in the figure were constructed using a special transcendental function called the Schottky-Klein prime function. Intruiguingly, they have been found to occur in a variety of problems in fluid dynamics.
Conformal mapping methods:
Read a recent review of "Conformal mapping methods for interfacial dynamics" which I wrote for Springer (with M. Bazant of MIT).
This is our winning entry to the ``Gallery of Nonlinear Images'' competition at the APS March Meeting in Canada in 2004. The simulation, performed using iterative conformal mapping techniques, is of transport-limited aggregation on the surface of a sphere (joint work with Bazant, Davidovitch and Choi).